MagicJigPipe
International Hazard
    
Posts: 1554
Registered: 19-9-2007
Location: USA
Member Is Offline
Mood: Suspicious
|
|
Calculus - Finding Critical Points & Factoring w/ Double Angle Formula
Okay, I am stuck on this one homework problem and perhaps you guys can help.
We must find critical points (min and max), points of inflection, intervals of concave up/down and intervals of decrease/increase of a particular
function. And then, of course, sketch the graph.
The function is f(x) = 2sin(x) + sin(2x)
I got the first derivative: f '(x) = 2cos(x) + 2cos(2x)
Now how do I use the double angle formula to factor this out? (and why does it work?)
The double angle formula says cos(2x) = 2cos^2(x) - 1
So,
f '(x) = 2cos(x) + (2)2cos^2(x) - 1
= 2cos(x) + 4cos^2(x) - 1
Now, how would I go about factoring that (so that I can set each factor equal to zero in order to get x values for the critical points)? Do I treat
4cos^2(x) - 1 as a single entity and if so, how? Is there an easier way to do this?
Thanks in advance.
[Edited on 11-10-2008 by MagicJigPipe]
"There must be no barriers to freedom of inquiry ... There is no place for dogma in science. The scientist is free, and must be free to ask any
question, to doubt any assertion, to seek for any evidence, to correct any errors. ... We know that the only way to avoid error is to detect it and
that the only way to detect it is to be free to inquire. And we know that as long as men are free to ask what they must, free to say what they think,
free to think what they will, freedom can never be lost, and science can never regress." -J. Robert Oppenheimer
|
|
|
UnintentionalChaos
International Hazard
    
Posts: 1454
Registered: 9-12-2006
Location: Mars
Member Is Offline
Mood: Nucleophilic
|
|
Ah, I wish my calculus class were anywhere near this easy anymore (god, I never thought I'd say that about any calculus but Im in calc 3 doing this
kinda stuff with three variables instead)
Just to explain the whole concept, your f(x) function, when fed an x value gives you a y variable. You plot a point on a graph. Now if you take the
derivative, you are finding an equation that when fed an x value spits out the rate of change in the y variable (the slope of the f(x) function) at
that point.
A critical point is a local minimum or maximum on your f(x) graph. It is the single infintesimally small spot at the bottom or top of a curve where
the tangent line is flat. If you are driving your car along and want to start driving backwards, there must be some point at which the car is neither
moving backward or forward. That's the critical point. since your car is stopped, the rate of change of your position (the derivative of your
position) is 0 miles per hour. If you set the derivative of a function equal to 0 and solve for the values of x, you will know where exactly your car
is stopped (assuming you can describe your bad driving in terms of a function). The double angle formula is just a handy trigonometric identity. The
derivation would probably confuse you more so just accept that it is true.
Your derivative is 2cos2x + 2cosx. Does that look like it would be easy to solve for x with? That's where the double angle formula comes in. wherever
you see cos2x, you insert 2((cosx)^2)-1, giving you:
0 = 2(2((cosx)^2)-1) + 2cosx
keep in mind that (cosx)^2 is the same as cos^2(x) and that it is equal to (cosx)(cosx). Distribute the previous equation giving:
0 = 4((cosx)^2)-2+2cosx = 4((cosx)^2)+2cosx-2
Now, for any given x (it will only be one value at a time), cosx is just a number and can be factored out or substituted for in the same way thel.
Lets swap it with u for a little bit to simplify:
0 = 4u^2+2u-2
See the common factor 2? divide the whole equation by two and it goes away:
0 = 2u^2+u-1
That should be pretty easy to factor now, no?
0 = (2u-1)(u+1)
Since anything multiplied by zero is zero, for the equation to be true, either
2u-1 = 0 --> u=1/2
or
u+1 = 0 --> u=-1
Now substitute cosx back in for u
cosx = 1/2 --> the cosine of what angle(s) is 1/2? Either pi/3 or 5pi/3.
Isn't it also true that an angle of 7pi/3 falls in the same spot as pi/3? So it should have the same cosine value, right? I got this angle by adding
2pi (one full revolution around the origin in radians). What if I subtract? Isn't -5pi/3 the same as pi/3 too? So we can express the answer as pi/3
+/- 2npi, with the stipulation that n is some integer (...-2,-1,0,1,2....) The same applies to 5pi/3 in notation.
cosx = -1 --> there is only one angle where this is true, and that is at pi. The same notation applies as above.
Hope that helps.
[Edited on 11-10-08 by UnintentionalChaos]
Department of Redundancy Department - Now with paperwork!
'In organic synthesis, we call decomposition products "crap", however this is not a IUPAC approved nomenclature.' -Nicodem
|
|
|
MagicJigPipe
International Hazard
    
Posts: 1554
Registered: 19-9-2007
Location: USA
Member Is Offline
Mood: Suspicious
|
|
Awesome, now I understand it. It was just the double angle substitution (and how to treat it) that was confusing me. Mostly because I talked to a
fellow classmate and she explained it horribly (usually I remember everything from trig but occasionally I'll just draw a blank).
Regardless, thanks for all of that!
You are a true "friend"!
I'm not working the rest of it right now as it's 4am and I just woke up temporarily (tomorrow afternoon is when I'll have time) but some others that I
talked to were having problems with the rest of the problem. But, I'll probably be able to do it...
Again, thanks.
I have Calc I, II, III and differential equations (or whatever the next one after III is called) to look forward to as well. I do know what you're
talking about. The old stuff looks SO easy compared to the new stuff. (even from last week) I heard that Calc II was the most difficult. Even
better, yes! *sarcasm* But seriously, I really do love learning.
It's late... tired.
Thanks...
[Edited on 11-10-2008 by MagicJigPipe]
"There must be no barriers to freedom of inquiry ... There is no place for dogma in science. The scientist is free, and must be free to ask any
question, to doubt any assertion, to seek for any evidence, to correct any errors. ... We know that the only way to avoid error is to detect it and
that the only way to detect it is to be free to inquire. And we know that as long as men are free to ask what they must, free to say what they think,
free to think what they will, freedom can never be lost, and science can never regress." -J. Robert Oppenheimer
|
|
|
MagicJigPipe
International Hazard
    
Posts: 1554
Registered: 19-9-2007
Location: USA
Member Is Offline
Mood: Suspicious
|
|
And now I see what I was doing wrong.
I was doing (2)2cos^2(x) - 1 instead of (2)(2cos^2(x) - 1). Now that I think about it some more it makes perfect sense. Since the 2 was multiplying
the entire cos(2x) then it should also be distributed across the entire substitution of 2cos^2(x) - 1.
Excellent.
My classmates are horrible at explaining things. They couldn't even explain that simple concept to me in person but you managed to do it through text
alone.
Thanks again.
"There must be no barriers to freedom of inquiry ... There is no place for dogma in science. The scientist is free, and must be free to ask any
question, to doubt any assertion, to seek for any evidence, to correct any errors. ... We know that the only way to avoid error is to detect it and
that the only way to detect it is to be free to inquire. And we know that as long as men are free to ask what they must, free to say what they think,
free to think what they will, freedom can never be lost, and science can never regress." -J. Robert Oppenheimer
|
|
|
MagicJigPipe
International Hazard
    
Posts: 1554
Registered: 19-9-2007
Location: USA
Member Is Offline
Mood: Suspicious
|
|
Okay, I've worked all the way through the problem but I am getting stuck on the last point of inflection. I have until 1pm today to finish this so I
would be so appreciative if you guys could help me before then.
Okay, picking up where I left off:
My domain is [0, 2π]
My critical points are:
(π/3, 2.598), (5π/3, -2.598), (π, 0), (2π, ~0), (0, 0) Do the 2pi and 0,0 count as critical points?
Increasing: [0, π/3)U(5π/3, 2π]
Decreasing: (π/3, 5π/3)
Got second derivative:
f ''(x) = -2sin(x) - 4sin(2x)
Then I used the double angle formula to get:
-2sin(x) - 8sin(x)cos(x) = 0
Then I divided all by -2:
sin(x) + 4sin(x)cos(x)
Which I then factored to:
sin(x)(4cos(x) + 1)
Then I set both factors equal to zero:
sin(x) = 0 4cos(x) + 1 = 0
Then I got:
sin(x) = 0 and cos(x) = -1/4 (not on unit circle damnit!)
Sine = 0 at pi and 2pi
I used arccos(-1/4) to get:
1.823 as my x value for that
Now I plugged these into the orginal function ( f(x)=2sinx + sin2x) to get their Y values which then gives the points of inflection:
(π, 0), (2π, 0), (1.823, 1.452)
But wait, I'm missing a point of inflection! How do I get the other point whose cosine equals -1/4 (besides 1.823)? I tried multiplying 1.823 by 2
but that doesn't seem to work. Or does it?
That's where I'm stuck pretty much. I realize that some of the zeros actually end up being like -2x10^-13 but I consider those close enough to zero
to be negligible in this situation.
Thanks in advance for the help! I appreciate this greatly.
"There must be no barriers to freedom of inquiry ... There is no place for dogma in science. The scientist is free, and must be free to ask any
question, to doubt any assertion, to seek for any evidence, to correct any errors. ... We know that the only way to avoid error is to detect it and
that the only way to detect it is to be free to inquire. And we know that as long as men are free to ask what they must, free to say what they think,
free to think what they will, freedom can never be lost, and science can never regress." -J. Robert Oppenheimer
|
|
|
UnintentionalChaos
International Hazard
    
Posts: 1454
Registered: 9-12-2006
Location: Mars
Member Is Offline
Mood: Nucleophilic
|
|
arccos on a calculator only gives one of the two values. Look at a unit circle. cosx = -1/4 should fall slightly past pi/2. Note that the cosine
values for the top and bottom of the unit circle are the same at any given x value. The answer the calculator spits out is the angle on the top
portion of the unit circle. To find its partner, subtract that number from 2pi.
The tiny values your calculator spit out are probably because of rounding and should actually equal zero.
For demonstrration purposes: Excuse the terrible image, but notice that the cos is the same at both angles. Also note that by themselves, theta1 is
the same number of radians as theta2. They only differ in which direction you came around the unit circle, either positive or negative.
[Edited on 11-11-08 by UnintentionalChaos]
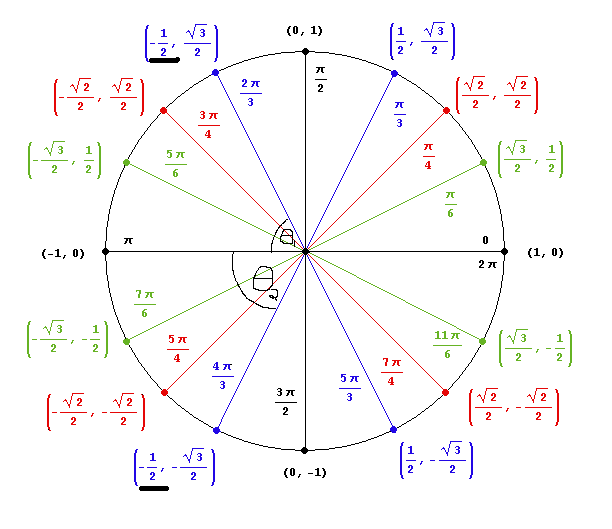
Department of Redundancy Department - Now with paperwork!
'In organic synthesis, we call decomposition products "crap", however this is not a IUPAC approved nomenclature.' -Nicodem
|
|
|
MagicJigPipe
International Hazard
    
Posts: 1554
Registered: 19-9-2007
Location: USA
Member Is Offline
Mood: Suspicious
|
|
Thanks so much. I did notice that, for example, 220* had the same cosine value as 110* (that might not be exact and I can't recall the equivalent in
radians but you get the gist I'm sure). However, in my haste I forgot to check if that applied to the rest of the values so I thought, "just multiply
it by two". But it just didn't seem right, so, there was my problem.
I tried a few other things though. Damn, I need to brush up on my trig. I took it during the summer (24 days of class in a month's time).
Because of you I have gained knowledge and possibly an A in the class (this "homework" counts as a lot; There were 6 of these problems so it took
hours).
Thanks so much for your help.
P.S. I need to make a unit circle that has 8 points (or more) in each quadrant instead of 4. The reason the unit circle falls on these numbers is to
avoid irrational numbers, right? But, then again, the chances of a number from a problem falling on one of the 4 new points would be slim... Okay,
I'm rambling. Bye.
[Edited on 2-20-2010 by Polverone]
"There must be no barriers to freedom of inquiry ... There is no place for dogma in science. The scientist is free, and must be free to ask any
question, to doubt any assertion, to seek for any evidence, to correct any errors. ... We know that the only way to avoid error is to detect it and
that the only way to detect it is to be free to inquire. And we know that as long as men are free to ask what they must, free to say what they think,
free to think what they will, freedom can never be lost, and science can never regress." -J. Robert Oppenheimer
|
|
|
woelen
Super Administrator
        
Posts: 8027
Registered: 20-8-2005
Location: Netherlands
Member Is Offline
Mood: interested
|
|
Also don't forget the cosine of pi/5. This also can be easily expressed. It equals (1+√5)/4.
|
|
|
GoatRider
Harmless

Posts: 21
Registered: 3-11-2008
Location: Minnesota
Member Is Offline
Mood: No Mood
|
|
| Quote: | Originally posted by woelen
Also don't forget the cosine of pi/5. This also can be easily expressed. It equals (1+√5)/4. |
That's my favorite. It's half the golden ratio.
|
|
|
JohnWW
International Hazard
    
Posts: 2849
Registered: 27-7-2004
Location: New Zealand
Member Is Offline
Mood: No Mood
|
|
| Quote: | Originally posted by woelen
Also don't forget the cosine of pi/5. This also can be easily expressed. It equals (1+√5)/4. |
The trigonometric ratios of angles which are multiples of ¶/17, ¶/257, and ¶/65537 can also be expressed as more complicated quadratic surds,
containing expressions such as 17 + sqrt(17) etc., by the solution of equations of the 8th, 128th, and 32,768th powers which reduce (through being
palindromic) to quadratic equations. These numbers, besides 3 and 5, are in the series 2^(2^n) + 1, called "Fermat primes". It was thought that these
numbers were all primes, until exceptions were found, the first being with n=641. I have the results for ¶/17 written down somewhere.
The trigonometric ratios of angles which are multiples of ¶/7 can be expressed as cubic surds containing "i" and sqrt(7) by solution of a cubic
equation. I also have these written down somewhere. The formulae for solutions of cubic equations can also be used as one-third-angle (trisection)
formulae, allowing the ratios of multiples of ¶/9 to be also expressed as cubic surds.
Of course, the trig ratios of multiples of ¶/8, ¶/16, ¶/32, ¶/64, ¶/12, ¶/24, ¶/48, ¶/20, ¶/40, etc., can be expressed as quadratic surds by
repeated application of the half-angle formulae. The trig ratios of multiples of ¶/15 etc. can be found as quadratic surds by the addition and
subtraction of angles formulae.
[Edited on 13-11-08 by JohnWW]
|
|
|
sparkgap
International Hazard
    
Posts: 1234
Registered: 16-1-2005
Location: not where you think
Member Is Offline
Mood: chaotropic
|
|
| Quote: | Originally posted by woelen
Also don't forget the cosine of pi/5. This also can be easily expressed. It equals (1+√5)/4. |
...and cos(2π/5) is half the reciprocal of the golden ratio. Fun. 
@JohnWW:
More trigonometric special values than what you can shake a slide rule at here and here. 
@MJP: When in the future you have these kind of problems that are best seen with a graph, this is helpful.
sparky (~_~)
sparky (~_~)
"What's UTFSE? I keep hearing about it, but I can't be arsed to search for the answer..."
|
|
|
MagicJigPipe
International Hazard
    
Posts: 1554
Registered: 19-9-2007
Location: USA
Member Is Offline
Mood: Suspicious
|
|
I knew what the graph looked like long before and I could get an approximate (x,y) coordinate but I needed to "show the work" that I used to get
there. So, simply plugging the function into my calculator and copying the values/graph wouldn't suffice.
However, that is an awesome site! I never knew that existed.
You know what (some people around my age may find this relevant)? I've always wondered why the TI-83 Graphing calculators have stayed the same
(technologically) for well over a decade and I think I've thought of a plausible answer. Could it be because, after many years of these calculators
not being accepted now that they finally are TI doesn't want to jeopardize this by changing the "format" or technology of it's calculators?
Does TI actually make graphing calculators that are on par with what computers of comparable size can do?
And, I wouldn't have a problem with any of this if they wouldn't keep charging the SAME DAMN PRICE. $130 for a "computer" with 32kb of RAM? Sh*t!
One could probably pay that much for an older PDA, add TI emulation software, and have a graphing calculator/Palm Pilot/MP3 Player/etc... for the same
price!
Okay, I'm done.
Thanks for all the help guys.
"There must be no barriers to freedom of inquiry ... There is no place for dogma in science. The scientist is free, and must be free to ask any
question, to doubt any assertion, to seek for any evidence, to correct any errors. ... We know that the only way to avoid error is to detect it and
that the only way to detect it is to be free to inquire. And we know that as long as men are free to ask what they must, free to say what they think,
free to think what they will, freedom can never be lost, and science can never regress." -J. Robert Oppenheimer
|
|
|
|