Rainwater
National Hazard
   
Posts: 919
Registered: 22-12-2021
Member Is Offline
Mood: indisposition to activity
|
|
Real gas law - van der Waals equation
So hyperspacepirate(youtube) is doing some cool stuff, and im trying to work out the math.
I've been able to rework the calculations for pressure and tempature easy enough, but for 2 days I tried to rework the algebra to solving for 'n'
until I googled it and read it cant be done algebraicly and requires iterative approximation.
Let me figure out how to write the math all pretty like
$$(P+\frac{an^2}{V^2})(V-nb)= nRT$$
So I punched in my values and equation into wolframalpha and got this nice little graph with 3 solutions.
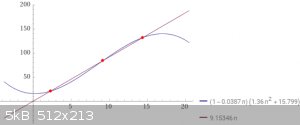
Can anyone elaborate on the subject?
At first glance, i would guess that these are values for three different phases(gas,liquid,solid).
For my project im trying to calculate how many grams of a gas is required to reach a desired partial pressure within a closed volume in a mixed gas
system.
To be more specific, a mixed refrigerant system.
As the first (higher boiling point) refrigerant is cooled enough to stay in liquid phase in the evaporator, it will reduce pressure in the condencer.
So I want to calculate how much of each refrigerant is required to maintain enough pressure to condence.
[Edited on 27-6-2023 by Rainwater]
"You can't do that" - challenge accepted
|
|
|
DraconicAcid
International Hazard
    
Posts: 4333
Registered: 1-2-2013
Location: The tiniest college campus ever....
Member Is Offline
Mood: Semi-victorious.
|
|
If you multiply it out, you get a third order polynomial for n. That will always have three solutions. it has nothing to do with three phases- it's
just math.
Just like a quadratic equation will have two solutions and a linear equation will have one solution.
Please remember: "Filtrate" is not a verb.
Write up your lab reports the way your instructor wants them, not the way your ex-instructor wants them.
|
|
|
Keras
National Hazard
   
Posts: 896
Registered: 20-8-2018
Location: (48, 2)
Member Is Offline
|
|
Quote: Originally posted by DraconicAcid  | | If you multiply it out, you get a third order polynomial for n. That will always have three solutions. it has nothing to do with three phases- it's
just math. |
Nah, cubic polynomials can have either 1 or 3 real roots. A real polynomial’s roots are always complex conjugates (easy to verify that if z is a
root, then z̅ is a root too), so they go in pairs, except when they are real (i.e. their own conjugates).
For example (z² + 1)(z - 1) = z³ - z² + z - 1 has only one real root, 1, and two more complex roots, ± i.
[Edited on 27-6-2023 by Keras]
|
|
|
Rainwater
National Hazard
   
Posts: 919
Registered: 22-12-2021
Member Is Offline
Mood: indisposition to activity
|
|
Do these 3 values give give the same pressure, temperature and volume?
Because that breaks my brain. What im seeing, mathematically. Ya, but no way.
Ok, dumb moment again. I see what i was doing wrong. So simple I missed it.
I wasnt solving for n, i was solving for nRT. About 4 days wasted
If I simplifie the equation to link
$$\frac{(P+\frac{an^2}{V^2})(V-nb)}{RT}= n$$
Then I still get 3 different answers, no way
Edit:using nitrogen gas at in this example, 111.55 kelvin, 15.799 atm
[Edited on 27-6-2023 by Rainwater]
To clarify, can anyone point me to an example that shows how the number of moles is calculated?
[Edited on 27-6-2023 by Rainwater]
"You can't do that" - challenge accepted
|
|
|
Keras
National Hazard
   
Posts: 896
Registered: 20-8-2018
Location: (48, 2)
Member Is Offline
|
|
Get a good book on physical chemistry. Atkin's is one of the best.
|
|
|
Rainwater
National Hazard
   
Posts: 919
Registered: 22-12-2021
Member Is Offline
Mood: indisposition to activity
|
|
Thank you for the reference.
So after some googleing, i discovered at those temps and pressures,
what van der waals is predicting is the phase transition to a liquid.
As more mass is added, it is changed into a liquid maintaining the pressure and tempature,
but now there is ratio of liquid/gas in the appratus
Essentially altering the volume and moles in the gas phase to maintain pressure and tempature until a critical point is reached when pressure must
increase
By increasing the temperature above the condensation point of the gas, only 1 solution is valid. As the temp approaches this point a range of
solutions become valid do to the liquid/vapor ratio
"You can't do that" - challenge accepted
|
|
|